1. 9 Lives Body Armor, Inc. produces and extremely unique type of body armor that is patented. 9 Lives is the only company in the world that possesses the technology to manufacture body armor that stops a bullet from literally any type of handgun, shotgun or rifle manufactured today. In addition to local, state, and federal law enforcement units, numerous private sector groups, individuals, and third world country have created a very high demand for this product.

The demand equation is given as   P = 2000 - .4Q
where Q is the annual sales quantity in suits of armor and P is the price per suit.
The firms total cost equation is   C = 900,000 + 250Q

a. To maximize profits, how many suits of armor should 9 Lives produce and sell?

What price should 9 Lives charge?

 
To maximize profits, set MR = MC

TR = P x Q = 2000Q - .4Q

MR = 2000 - .8 Q

MC = 250

2000 - .8Q = 250

Q = 2,187.5….. Q = 2,187

P =2000 - .4(2,187) = 2000 - 875 = $1,125

P = $1,125, Q = 2,187

2. Compute the total profit for 9 Lives in this monopoly environment.

 
P = rev - cost = [2000(2187) - .4(2187)­ 2] - [900,000 - 250(2187)]

4374000 - 1913187 - 900000 - 546750

 
Õ = $1,014,062

 
The US Government quickly grows fearful that this type of armor will threaten national security. It immediately limits production of this armor to 1,000 suits per year and implements a serial number registration system so the government can track the users of this equipment.

3. What effect does this have on price and profit for 9 Lives?

P = 2000 - .4(1,000)

P = 1,600 per suit

Õ = [2000(1000) - .4(1000)­ 2] - [900000 + 250(1000)]

• = $450,000

Note that although the regulated production leads to a higher price, profits are reduced because the optimal price is lower. Higher prices do not mean higher profits.
 

4. Based on the decrease in profits due to government intervention, should the government offer some type of subsidy to 9 Lives to make up the loss?

No. In an ordinary free-market society, one's initial reaction may be to say that

the Government should reimburse this company for the loss in profits. Due to the

nature of the business, however, it is in the publics best interest for the

Government to regulate this type of product. There are many illegal products

that would be extremely profitable in today’s economy if they were allowed to be

sold on the free market, but those products are illegal for a reason and that is for

the benefit of the general public and the welfare of the nation. While this product

was not declared "illegal", it was recognized to pose a significant risk to the

ability of our law enforcement/military personnel to control the actions of

potentially dangerous people that might use this product to enable them to

conduct illegal activities without the fear of deadly force being used to stop them.

Note: In addition to regulation, the government must impose some enforcement mechanism. 9 lives would like like to expand production and lower prices, and there are willing buyers. There is thus the possibility of "black markets" and illicit production.

2. McMurphy is one of a handful of OU students that are currently manufacturing and selling home brew beer on campus. McMurphy's business is in a monopolistcally competitive market and his weekly demand and cost functions are as follows:
 

P=10-.25Q and C=50+.5Q

 
a. What are McMurphy's optimum short term price, quantity and profits?

 
MR=10-.5Q MC=.5

10-.5Q=.5

Q=19 and P=5.25

P =TR-C=99.75-59.5=40.25
 

b. It seems likely that other students will also find it profitable to make their home brews for sale. What should McMurphy expect his long term equilibrium price and quantity to be?

 
AC=(50+.5Q)/Q

 
dp/dq=dac/dq (The demand must be tangent to AC as a result of entry)

 
-.25=-50/Q2

Q=14

AC=P

P=(50+.5(14))/14=4.07

 
c. Can this market becomes perfectly competitive? If so, determine the long-run equilibrium price and quantity.

In perfect competition, price must go to the minimum of the AC. With a U-shaped cost curve, find the minimum by in the usual way taking the derivative of AC. Here, however, the AC is not U-shaped; MC is constant at 0.5. Thus the market is incompatible with a firm being a price-taker (i.e., both the MC and MR would be horizontal).

3. It is the year 2050 and the market for lunar travel (spaceship from earth to the moon) is extremely regulated. The barriers to entry of new spaceship companies are enormous, and flight fares are set by regulation. Suppose that the annual lunar travel demand is estimated to be Q=300000-5P(or P=60000-Q/5), where Q is the number of trips in thousands and P is theone-way fare in dollars. (For example, 250000 annual trips are taken when the fare is $10,000). Also, the long-run average (one way) cost per passenger is estimated to be $6000.

250 - 2(Q_CAF + Q_AM) = 24 + 6Q_CAF = 10 + 2Q_AM

Q_CAF = Q_AM

250 - 4Q = 24 -6Q = 10 + 2Q

Q_CAF + Q_AM = 20

This MR of 210 exceeds the MC of both firms so by expanding production to 30 units CAF Corporation can increase its profits. In the long run however this strategy will not work. AM Corporation will want to increase its output it match the profits of CAF Corporation and this competition will damage the cartel and ultimately destroy the cartel.

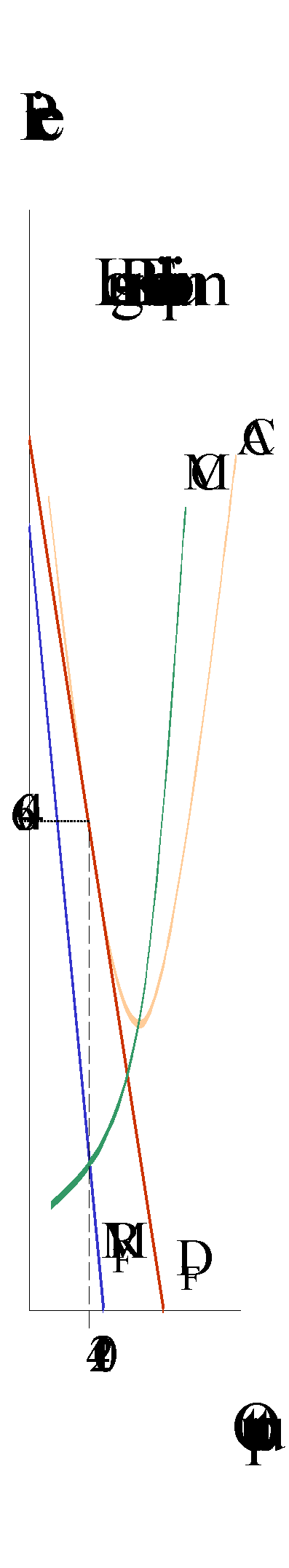
5. The Starhopper Corporation has created the first and only transportation vehicle designed for interplanetary space travel with a revolutionary, low cost, power source. The demand function is as follows:

Q = f (P, E, S, A, PS, C)

Due to current news reports on near asteroid collisions the demand equation is large and has the following formula:

Q_D = 456008 - 0.35P + 4.76E + 75.86S + 2456A + 57.6PS + 36.7C

a. For the last four months, the Starhopper Corporation has been selling their StarM5 vehicle. They are a monopoly and have acquired the patents necessary to remain so for the near future. The current economy is good at 5.2, current safety concerns are wavering at 74, the current news has been talking of nothing else the last few weeks (67 pts), most persons are able to withstand space travel (89%) and the power supply is projected to last for the next millenium (1000 years). The short run cost per vehicle is as follows:

STC = 3455400 + 250Q + 764.3Q²

In order to maximize their profit, how many StarM5's should they plan to produce? At what price? What will be the profit?

Q_D = 683,831.055 - .0.35P Þ P = 1,953,803 - 2.857Q

Q = 1273.24 Þ 1273

p = R - C = 2,482,561,318 - 1,242,343,965 = $1,240,217,353

b. Because of the revolutionary new power source that Starhopper has created using Methane gas, it now falls within the category of a natural monopoly and the long run average cost is declining to the following equation:

LAC = 1714.52 - 0.568 - 0.45892Q

Q

What is the new quantity, price and profit?

Q = 383688.566 Þ 383688

p = R - C = (P x Q) - C = 3.2905313 x 10¹¹ - (-6.7560803 x 10¹⁰ ) = 3.9661393 x 10¹¹

Þ $396,613,930,000

c. The government decides to regulate this industry and initiates average-cost pricing. Under this new policy what is the new quantity and price?

1,953,803 - 2.857Q = 1714.52 - 0.568 - 0.45892Q

Using the quadratic equation: - b ± Ö b² - 4ac

2a

Q = - 1,953,803.568 ± Ö 1,953,803.568² - 4 (-2.39808) (1714.52)

2(-2.39808)

d. Scientists at Starhopper are worried that NASA will decide that it can also get in on the profits and chooses to enter into competition. As NASA would have to develop a different power source (due to patents) their costs would be a bit higher but their reputation should earn them close to the following demand and cost equations:

Q_NASA = 6890210 - 0.35P + 4.76E + 75.86S + 2456A + 265PS + 36.7C

All factors should stay the same except that their probable power supply is expected to last only 150 years, therefore: Q_NASA = 898,994 - 0.35P P_NASA = 2,568,554 - 2.857Q

Is NASA a potential threat? What are its expected quantities and price?

Q_D = 1,100,183 - .0.35P Þ P = 3,143,380.157- 2.857Q

Q = 2089.317 Þ 2089

e. Assuming a perfectly competitive situation, the industry demand and supply curves are expected to be the following:

Q = 449,497 - 0.35P S = MC = 333 + 756.8Q

What would the new price be for both companies in a perfectly competitive market?

f. Determined not to get caught in this situation and hearing rumors that NASA is contemplating entering the industry, Starhopper proposes forming a cartel with NASA. Will NASA agree?

g. In the event that a cartel can not be formed, what other options is open to Starhopper to dissuade competition?

6. Tortilla Bell is a new restaurant. Based on the fact that it has a unique menu and waitresses with special uniforms, the restaurant commands a premium price. In the short run, the demand curve and cost equations for a Premium Tortilla Plate per day are:

P = 8 - .025Q C = 100 + 4Q

a. What are the short term, profit-maximizing output, price, and profit?

b. Determine the long run price and output assuming that the demand curve shifts in a parallel manner.

c. What can Tortilla Bell do to again start making economic profits?

1. Q = 2020 – 98P Demand equation

P = 20.61 - .0102Q Inverse Demand Equation

TR = (20.61 - .0102Q)Q

TR = 20.61Q - .0102Q² Total Revenue Equation

MR = 20.61 - .0204Q Marginal Revenue Equation

TC = 2300 + 2.25Q Total Cost Equation

MC = 2.25 Marginal Cost

 

MR = MC 900 = 2020 – 98P

20.61 - .0204Q = 2.25 98P = 1120

18.36 = .0204Q P = $ 11.43

Q = 900

 

TP = TR – TC

= (900 x 11.43) – [2300 + (2.25 x 900)]

TP = $ 5962

 

 

2. Q = 1678 - 105P Demand equation

P = 15.98 - .0095Q Inverse Demand Equation

TR = (15.98 - .0095Q)Q

TR = 15.98Q- .0095Q² Total Revenue Equation

MR = 15.98- .019Q Marginal Revenue Equation

TC = 2300 + 2.25Q Total Cost Equation

MC = 2.25 Marginal Cost

 

MR = MC 722 = 1678 – 105P

15.98- .019Q = 2.25 105P = 956

13.73 = .019Q P = $ 9.10

Q = 722

 

TP = TR – TC Quantity decreased by 178

= (722 x 9.10) – [2300 + (2.25 x 722)] Price decreased by $2.24

TP = $ 2645.70 Total Profits decreased by $3316.30

 

 

 

3. Assuming that Barnstormers!’ demand is the market demand, the restaurant simply sets MR = MC to derive its optimal price and output.

 

 

4. We need dP/dQ=dAC/dQ assuming a parallel shift in demand until it is tangent to the AC.